Single DOF Dynamics - Spring with attached mass.xls
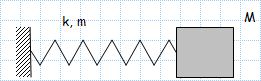
Description
Calculate natural frequency of coil spring with mass attached.
Calculation Reference
Harris' Shock and Vibration Handbook
A single degree of freedom (DOF) dynamic system consists of an object that can move in only one direction, with its motion being described by a single coordinate, usually displacement. One of the simplest examples of a single DOF system is a mass attached to a spring, which can oscillate along a straight line.
In this system, the mass is connected to the spring, and the spring is fixed to a stationary point. When the mass is displaced from its equilibrium position and then released, it experiences a restoring force exerted by the spring. According to Hooke's law, the restoring force is proportional to the displacement of the mass and acts in the opposite direction of the displacement. The equation representing Hooke's law is:
F = -k * x
where:
- F is the restoring force
- k is the spring constant, a measure of the stiffness of the spring
- x is the displacement from the equilibrium position
The motion of the mass is governed by Newton's second law of motion, which relates the mass's acceleration to the net force acting on it:
m * a = F
where:
- m is the mass of the object
- a is the acceleration
For a spring-mass system, combining Newton's second law and Hooke's law yields the following differential equation:
m * x''(t) = -k * x(t)
where:
- x(t) is the displacement as a function of time (t)
- x''(t) is the second time derivative of x(t), representing acceleration
The solution to this differential equation is a sinusoidal function, which describes the simple harmonic motion of the mass:
x(t) = A * cos(ωt + φ)
where:
- A is the amplitude of the oscillation, representing the maximum displacement from the equilibrium position
- ω (omega) is the angular frequency, related to the natural frequency (f_n) of the system by ω = 2π * f_n. The natural frequency is determined by the mass and the spring constant: f_n = (1 / 2π) * √(k / m)
- φ (phi) is the phase angle, which depends on the initial conditions of the system
The single DOF spring-mass system is a fundamental model in the study of vibrations and dynamics, as it helps to understand the basic principles of oscillatory motion and the behavior of more complex systems.
Calculation Preview
Full download access to any calculation is available to users with a paid or awarded subscription (XLC Pro).
Subscriptions are free to contributors to the site, alternatively they can be purchased.
Click here for information on subscriptions.

